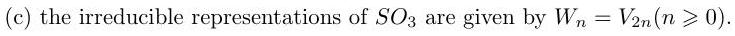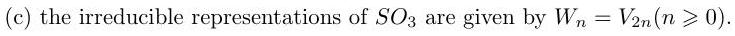Let Vn be the space of homogeneous polynomials of degree n in two variables z1 and z2. Define a left action of G=SU2 on the space of polynomials by setting
(gP)z=P(zg),
where P∈C[z1,z2],g=(acbd),z=(z1,z2) and zg=(az1+cz2,bz1+dz2).
Show that
(a) the representations Vn are irreducible,
(b) the representations Vn exhaust the irreducible representations of SU2, and