Paper 1, Section I, D
Part II, 2015
(a) The action for a one-dimensional dynamical system with a generalized coordinate and Lagrangian is given by
State the principle of least action and derive the Euler-Lagrange equation.
(b) A planar spring-pendulum consists of a light rod of length and a bead of mass , which is able to slide along the rod without friction and is attached to the ends of the rod by two identical springs of force constant as shown in the figure. The rod is pivoted at one end and is free to swing in a vertical plane under the influence of gravity.
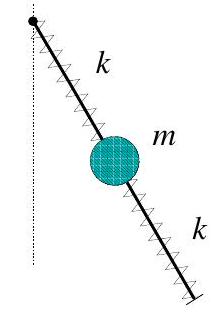
(i) Identify suitable generalized coordinates and write down the Lagrangian of the system.
(ii) Derive the equations of motion.